Measuring the distance between stars

  

    The universe is one of the vast things ever we can imagine. There are millions of galaxies in the universe which has trillions of stars, planets, and gaseous components in them. One of the most primary objects in every galaxy is the star. 

Credit: By ESA/Hubble, CC BY 4.0, https://commons.wikimedia.org/w/index.php?curid=8788068

    Each star is a huge, hot glowing plasma, emitting a brilliant light from it. Each star is located at a very vast distance from the other. They are very far, that the largest star ever we know, the UY Scuti, which is a thousand times bigger than our sun, appears as a small dot, even when observed through a telescope from the earth. Because this star is around 5,219 light-years away, which is 30,680,823,987,910,600 miles or 49,376,000,000,000,000 (above 49 quadrillion) km away; which is a huge distance. 

 

Credit: By Philip Park - Own work, CC BY-SA 3.0, https://commons.wikimedia.org/w/index.php?curid=31016689

    A light-year is a type of unit which is used to measure enormous distances. As the speed of the light is 299,792,458 miles/sec and it covers 9.264*10¹² km in one year (popularly called one light-year), it could cover a large distance in a quick period of time. Thus measuring such a long distance by kilometres and miles might not be a suitable idea, which would take a huge number for calculation as seen above. So light years are used to make the calculation simple. Our neighbouring star Proxima Centauri is located at 4.26 light-years, which means if the light is passed from the earth to the Proxima Centauri, it takes 4.26 years to reach that star. So the light we are currently seeing now from the star Proxima Centauri is 4.26 light-years back. Suppose if the star Proxima Centauri died now, we will be able to notice it only after 4.26 years. Even the light from our sun, which is 149.6 million away from us, takes about 8 minutes and 20 seconds to reach earth. So that the light we are currently seeing now from the sun is 8 minutes and 20 seconds back. So to measure the timing between the celestial bodies, the distance between them has to be measured. But how scientists figure out the distance? Let’s find out. 

Light moving speed between the Earth and the Moon in real-time

     There are several ways in which the distance between the stars can be found. The first method the scientists discovered to find the distance between the stars is the trigonometric parallax. A parallax is an effect in which the position of an object will seem to be changed when viewed from different positions. It can also be described as; when we are moving; the nearer objects will appear to be moving faster while far objects appear to be moving slower. 

    For example, when we stretch our hand and see our thumb by alternatively closing our eyes, our thumb will seem to be moving each time when we close our eyes. By using this distance between our eyes and the shifted angle of our finger we can figure out the distance from our thumb to our eye. So by using the same method and replacing our thumb with the star, the distance can be found to the nearby star. But as an object moves further from our eye, the movement seems to be hard to detect. The reason we cannot detect the movement is the same reason we have seen above, that when moving, objects nearer will move faster while objects further will move slower. As a star is at a very far distance we cannot detect the movement by simply closing our eyes. So by increasing the baseline, which is the distance between our two eyes, we can able to notice the movement. So we choose the whole earth itself as an eye. At one point of the year, we look at the star and measure its angle by marking the path to the star. Again after six months, we look at the same star and measure its angle; because during this duration of these six months, the earth would have completed half of its orbit. Now after the six months, if we observe the star, it appears to be moved through some distance from the previous point. By knowing the distance between the sun and the earth which is 149.6 million km (The Astronomical Unit) and the shifted angle of the star we can easily find the distance.

   Now let’s take a star as an example whose distance has to be found. We can only able to notice the parallax effect if an object behind the star is stationary. If the object behind the star is moving, we would not be able to notice the change of position of the star with respect to the earth’s position. Now we make two angle measurements over the span of six months by the different positions of the earth. By taking these two angles we can find out the distance to the star.

Parallax Method

 

    We know that the sky is 360°. If we split that each degree, we get 60 arc minutes, and again if we split each arc minute we get 60 arc seconds. It is the same as hours split into minutes and minutes split into seconds. Let’s say that the angle we measured in the sky over a course of six months is one arc second. Now by taking one right-angled triangle and applying some trigonometry we can find out the distance.

Relation between Degree, Arc Minute, and Arc Second

Let’s first take,

                                         tanθ =opposite side/adjacent side

     Here the opposite side will be the distance (d) between the sun and the star to be found. The adjacent side will be the distance between the Earth and the Sun, which is One Astronomical Unit (1 AU). So the equation becomes,

                                       tanθ= d/1AU

    To find the tangent term, we need to find the value of Θ. The value of Θ is the angle we measured. In a right-angled triangle, the maximum degree is 90°. But this value of the degree is reduced by one arc second as shown in the diagram above, so the value of Θ becomes (90°-Θ). By substituting this value, the equation becomes,

                                      tan (90°-θ) =d/1AU

 Now the Θ value becomes 1 arc second which is 1/3600^th of a degree

                      So,          tan (90-(1/3600)) =d/1AU

By solving the equation, we get,

                                     206,265*1AU=d

    Therefore d=206265 AU which is approximately 1 Parsec away. So, 1 Parsec is defined as the parallax angle of one arc second. If we want to convert this Astronomical value into light-years, we proceed with,

                                      1 light-year = 63241.077AU

So,                                206,265 AU= 206,265/63241.077 light years

 Which is,                                          =3.26 light-years.

   So the distance to the star from the sun is 206,265 AU or 1Parsec or 3.26 light-years. And that’s how scientists figured out the distance between the stars. But this method is possible for the measurement of stars up to 30.66 Parsecs. Above that, the parallax angle would become too tiny to detect and we will be unable to find the distance to the star. So the distance to the star which is above 30.66 Parsecs can be found by an amazing method called Standard Candles. Let’s see what this Standard Candle is,

   Standard Candle is the term which is indicating the term luminosity (light intensity) that is well known. So the star, whose brightness was well known, can be used as standard candles. First, if we know the intrinsic brightness of the star (its full brightness) and the value of brightness we get at a certain extent, we can find the amount of distance, the star is been.

  

Pulsing rate of Cepheid Variables

    There is a special type of star which is commonly used as a standard candle for measuring most distant objects in the universe. These stars are known as Cepheid variables, which are potentially unstable, that the light emitted by the star varies with the standard period of time, which means that the star exhibits its maximum brightness at one point and slowly lowers its brightness and again becomes brighten at another point with a standard period of time. So by measuring the period of time (i.e., the time from its full brightness to emit its full brightness again), we can find the distance of very far away objects.

Credit (Cepheid Variable): By Merikanto - Own work, CC BY-SA 4.0, https://commons.wikimedia.org/w/index.php?curid=79918260

 

Area covered by light with respect to distance

    First, the distance of the nearest Cepheid Variable star is known by using the parallax method. Then we have to know the light we have got in flux per meter square. We are measuring the light in flux/m², because the light emitted from a source always keeps on expanding by travelling outwards. So the light, receiving our telescope is only a part of the light emitted by the star. So we measure the flux value in m². So the brightness decreases with the square in the distance. Once the distance and the flux value was known, we can find the total luminous of the object, by using the formula,

F=L/4πr²

Here F is the flux value in m².

L is the total luminosity of the object.

r² is the square of the distance from the earth to the star, according to the light emitted by the star.

   Here the area of the sphere is used because all the stars in the universe appear to be spherical in nature. So the light emitted by the star depends upon the average area of the star. So by knowing the area, got by substituting the distance value obtained from the parallax method we can find how luminous the object is. Thus we can confirm that the star which is present at this distance and this period of change (from full brightness to again full brightness) has this amount of luminous. Suppose if we have to find the distance of any other star which has the same period of deviation, and which cannot be found by the parallax method, we can simply calculate the change in the intensity of the light and we can able to calculate the distance of that star also.  Now if we found the distance of the new star we can substitute in the above flux formula and we can able to find how actual luminous it was. So now by keeping it as a base we can precede our calculation to more far stars.   

Type 1a Supernova

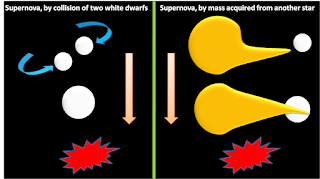
    But even Cepheid variables can be observed only for a certain distance. Below that point, it will be unnoticeable. So, scientists figured out another way to measure even more distant objects. That method is called a supernova. Supernova is a huge blast that occurs in space when huge massive stars die. Supernovae are of various types, and the main type of supernova used in measuring distance is the Type 1a Supernova.  This Type 1a supernova occurs when a white dwarf collapses by absorbing mass from another source or when another white dwarf comes near to it. This supernova can last for many days and even for months. And it is visible even from other galaxies, which makes it perfect for measuring far objects. So by knowing the luminosity of the star which is near to the supernova and by using the parallax and the flux calculation method, we can find the distance to the supernova depending upon the period of maximum brightness. So again by calculating its distance we can find how luminous it was and we can find the distance of even more far objects. There are still more methods to find the distance to very distant objects, which can be seen in the below image. This is how scientists figured out the distance to these most beautiful objects in this amazing universe.

Different measuring systems for longer distances